Question.
In figure, (i) and (ii), $\mathrm{DE} \| \mathrm{BC}$. Find $\mathrm{EC}$ in (i) and $\mathrm{AD}$ in (ii).
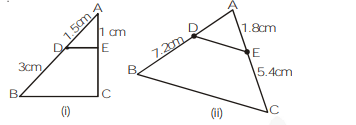
In figure, (i) and (ii), $\mathrm{DE} \| \mathrm{BC}$. Find $\mathrm{EC}$ in (i) and $\mathrm{AD}$ in (ii).

Solution:
(i) In figure, (i) DE $\| \mathrm{BC}$ (Given)
$\Rightarrow \frac{A D}{D B}=\frac{A E}{E C}$ (By Basic Proportionality Theorem)
$\Rightarrow \frac{1.5}{3}=\frac{1}{E C}$
$\{\because \mathrm{AD}=1.5 \mathrm{~cm}, \mathrm{DB}=3 \mathrm{~cm}$ and $\mathrm{AE}=1 \mathrm{~cm}\}$
$\Rightarrow \mathrm{EC}=\frac{3}{1.5}=2 \mathrm{~cm}$
(ii) In fig. (ii) $D E \| B C$ (given)
So, $\frac{A D}{B D}=\frac{A E}{C E} \Rightarrow \frac{A D}{7.2}=\frac{1.8}{5.4}$
$\{\because \mathrm{BD}=7.2, \mathrm{AE}=1.8 \mathrm{~cm}$ and $\mathrm{CE}=5.4 \mathrm{~cm}\}$
$\mathrm{AD}=2.4 \mathrm{~cm}$
(i) In figure, (i) DE $\| \mathrm{BC}$ (Given)
$\Rightarrow \frac{A D}{D B}=\frac{A E}{E C}$ (By Basic Proportionality Theorem)
$\Rightarrow \frac{1.5}{3}=\frac{1}{E C}$
$\{\because \mathrm{AD}=1.5 \mathrm{~cm}, \mathrm{DB}=3 \mathrm{~cm}$ and $\mathrm{AE}=1 \mathrm{~cm}\}$
$\Rightarrow \mathrm{EC}=\frac{3}{1.5}=2 \mathrm{~cm}$
(ii) In fig. (ii) $D E \| B C$ (given)
So, $\frac{A D}{B D}=\frac{A E}{C E} \Rightarrow \frac{A D}{7.2}=\frac{1.8}{5.4}$
$\{\because \mathrm{BD}=7.2, \mathrm{AE}=1.8 \mathrm{~cm}$ and $\mathrm{CE}=5.4 \mathrm{~cm}\}$
$\mathrm{AD}=2.4 \mathrm{~cm}$
Click here to get exam-ready with eSaral
For making your preparation journey smoother of JEE, NEET and Class 8 to 10, grab our app now.
