Question.
In figure, $\mathrm{A}, \mathrm{B}$ and $\mathrm{C}$ are points on $\mathrm{OP}, \mathrm{OQ}$ and $\mathrm{OR}$ respectively such that $\mathrm{AB} \| \mathrm{PQ}$ and $\mathrm{AC}$ $\| \mathrm{PR}$. Show that $\mathrm{BC} \| \mathrm{QR}$.
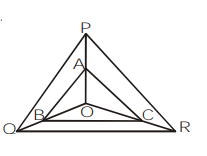
In figure, $\mathrm{A}, \mathrm{B}$ and $\mathrm{C}$ are points on $\mathrm{OP}, \mathrm{OQ}$ and $\mathrm{OR}$ respectively such that $\mathrm{AB} \| \mathrm{PQ}$ and $\mathrm{AC}$ $\| \mathrm{PR}$. Show that $\mathrm{BC} \| \mathrm{QR}$.

Solution:
In $\triangle \mathrm{POO}$
AB $\| P Q$ (given)
$\frac{\mathrm{OB}}{\mathrm{BQ}}=\frac{\mathrm{OA}}{\mathrm{AP}} \ldots$ (i) (Basic Proportionality Theorem)
In $\triangle \mathrm{POR}$
$\mathrm{AC} \| \mathrm{PR}$ (given)
$\frac{\mathrm{OA}}{\mathrm{AP}}=\frac{\mathrm{OC}}{\mathrm{CR}} \ldots$ (ii) (Basic Proportionality Theorem)
From (i) and (ii), we get
$\frac{\mathrm{OB}}{\mathrm{BQ}}=\frac{\mathrm{OC}}{\mathrm{CR}}$
$\therefore$ By converse of Basic Proportionality Theorem,
$B C \| O R$
In $\triangle \mathrm{POO}$
AB $\| P Q$ (given)
$\frac{\mathrm{OB}}{\mathrm{BQ}}=\frac{\mathrm{OA}}{\mathrm{AP}} \ldots$ (i) (Basic Proportionality Theorem)
In $\triangle \mathrm{POR}$
$\mathrm{AC} \| \mathrm{PR}$ (given)
$\frac{\mathrm{OA}}{\mathrm{AP}}=\frac{\mathrm{OC}}{\mathrm{CR}} \ldots$ (ii) (Basic Proportionality Theorem)
From (i) and (ii), we get
$\frac{\mathrm{OB}}{\mathrm{BQ}}=\frac{\mathrm{OC}}{\mathrm{CR}}$
$\therefore$ By converse of Basic Proportionality Theorem,
$B C \| O R$
Click here to get exam-ready with eSaral
For making your preparation journey smoother of JEE, NEET and Class 8 to 10, grab our app now.
