Question.
In figure, $\mathrm{ABC}$ and $\mathrm{DBC}$ are two triangles on the same base $\mathrm{BC}$. If $\mathrm{AD}$ intersects $\mathrm{BC}$ at $\mathrm{O}$, show that $\frac{\operatorname{ar}(A B C)}{\operatorname{ar}(D B C)}=\frac{A O}{D O}$.'
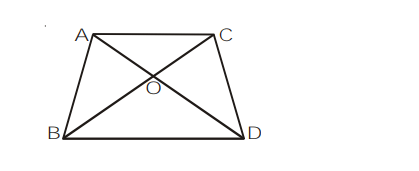
In figure, $\mathrm{ABC}$ and $\mathrm{DBC}$ are two triangles on the same base $\mathrm{BC}$. If $\mathrm{AD}$ intersects $\mathrm{BC}$ at $\mathrm{O}$, show that $\frac{\operatorname{ar}(A B C)}{\operatorname{ar}(D B C)}=\frac{A O}{D O}$.'

Solution:
Draw $\mathrm{AL} \perp \mathrm{BC}$ and $\mathrm{DM} \perp \mathrm{BC}$ (see figure)
$\Delta \mathrm{OLA} \sim \Delta \mathrm{OMD}$(AA similarity criterion)
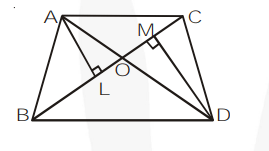
$\Rightarrow \frac{A L}{D M}=\frac{A O}{D O}$ ...(1)
Now, $\quad \frac{\operatorname{ar}(\Delta A B C)}{\operatorname{ar}(\Delta D B C)}=\frac{\frac{1}{2} \times(B C) \times(A L)}{\frac{1}{2} \times(B C) \times(D M)}$
$=\frac{\mathrm{AL}}{\mathrm{DM}}=\frac{\mathrm{AO}}{\mathrm{DO}}$ (by1)
Hence, $\frac{\operatorname{ar}(\Delta A B C)}{\operatorname{ar}(\Delta D B C)}=\frac{A O}{D O}$
Draw $\mathrm{AL} \perp \mathrm{BC}$ and $\mathrm{DM} \perp \mathrm{BC}$ (see figure)
$\Delta \mathrm{OLA} \sim \Delta \mathrm{OMD}$(AA similarity criterion)

$\Rightarrow \frac{A L}{D M}=\frac{A O}{D O}$ ...(1)
Now, $\quad \frac{\operatorname{ar}(\Delta A B C)}{\operatorname{ar}(\Delta D B C)}=\frac{\frac{1}{2} \times(B C) \times(A L)}{\frac{1}{2} \times(B C) \times(D M)}$
$=\frac{\mathrm{AL}}{\mathrm{DM}}=\frac{\mathrm{AO}}{\mathrm{DO}}$ (by1)
Hence, $\frac{\operatorname{ar}(\Delta A B C)}{\operatorname{ar}(\Delta D B C)}=\frac{A O}{D O}$
Click here to get exam-ready with eSaral
For making your preparation journey smoother of JEE, NEET and Class 8 to 10, grab our app now.
