In figure, if DE || BC,

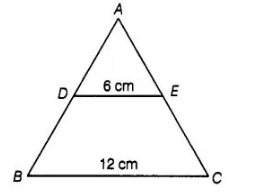
Given, $D E \| B C, D E=6 \mathrm{~cm}$ and $B C=12 \mathrm{~cm}$ In $\triangle A B C$ and $\triangle A D E$,

$\angle A B C=\angle A D E$ [corresponding angle]

$\angle A C B=\angle A E D$ [corresponding angle]

$\begin{array}{lll}\text { and } & \angle A=\angle A & \text { [common side] }\end{array}$

$\therefore \quad \triangle A B C \sim \triangle A E D \quad$ [by AAA similarity criterion]

Then, $\frac{\operatorname{ar}(\Delta A D E)}{\operatorname{ar}(\Delta A B C)}=\frac{(D E)^{2}}{(B C)^{2}}$

$=\frac{(6)^{2}}{(12)^{2}}=\left(\frac{1}{2}\right)^{2}$

$\Rightarrow$ $\frac{\operatorname{ar}(\Delta A D E)}{\operatorname{ar}(\Delta A B C)}=\left(\frac{1}{2}\right)^{2}=\frac{1}{4}$

Let $\operatorname{ar}(\Delta A D E)=k$, then $\operatorname{ar}(\Delta A B C)=4 k$

Now, ar $(D E C B)=\operatorname{ar}(A B C)-\operatorname{ar}(A D E)=4 k-k=3 k$

$\therefore$ Required ratio $=\operatorname{ar}(A D E): \operatorname{ar}(D E C B)=k: 3 k=1: 3$