In figure, if $\mathrm{LM} \| \mathrm{CB}$ and $\mathrm{LN} \| \mathrm{CD}$, prove that $\frac{\mathrm{AM}}{\mathrm{AB}}=\frac{\mathrm{AN}}{\mathrm{AD}}$.
Question.
In figure, if $\mathrm{LM} \| \mathrm{CB}$ and $\mathrm{LN} \| \mathrm{CD}$, prove that $\frac{\mathrm{AM}}{\mathrm{AB}}=\frac{\mathrm{AN}}{\mathrm{AD}}$.
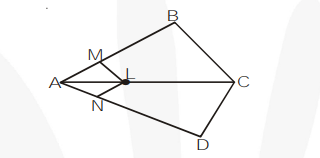
In figure, if $\mathrm{LM} \| \mathrm{CB}$ and $\mathrm{LN} \| \mathrm{CD}$, prove that $\frac{\mathrm{AM}}{\mathrm{AB}}=\frac{\mathrm{AN}}{\mathrm{AD}}$.

Solution:
In $\triangle \mathrm{ACB}$ (see figure), $\mathrm{LM} \| \mathrm{CB}$ (Given)
$\Rightarrow \frac{A M}{M B}=\frac{A L}{L C}$ ...(1)
(Basic Proportionality Theorem)
In $\triangle \mathrm{ACD}$ (see figure), $\mathrm{LN} \| \mathrm{CD}$ (Given)
$\Rightarrow \frac{A N}{N D}=\frac{A L}{L C}$ ...(2)
(Basic Proportionality Theorem)
From (1) and (2), we get
$\frac{A M}{M B}=\frac{A N}{N D}$
$\Rightarrow \frac{\mathrm{AM}}{\mathrm{AM}+\mathrm{MB}}=\frac{\mathrm{AN}}{\mathrm{AN}+\mathrm{ND}} \Rightarrow \frac{\mathrm{AM}}{\mathrm{AB}}=\frac{\mathrm{AN}}{\mathrm{AD}}$
In $\triangle \mathrm{ACB}$ (see figure), $\mathrm{LM} \| \mathrm{CB}$ (Given)
$\Rightarrow \frac{A M}{M B}=\frac{A L}{L C}$ ...(1)
(Basic Proportionality Theorem)
In $\triangle \mathrm{ACD}$ (see figure), $\mathrm{LN} \| \mathrm{CD}$ (Given)
$\Rightarrow \frac{A N}{N D}=\frac{A L}{L C}$ ...(2)
(Basic Proportionality Theorem)
From (1) and (2), we get
$\frac{A M}{M B}=\frac{A N}{N D}$
$\Rightarrow \frac{\mathrm{AM}}{\mathrm{AM}+\mathrm{MB}}=\frac{\mathrm{AN}}{\mathrm{AN}+\mathrm{ND}} \Rightarrow \frac{\mathrm{AM}}{\mathrm{AB}}=\frac{\mathrm{AN}}{\mathrm{AD}}$
Click here to get exam-ready with eSaral
For making your preparation journey smoother of JEE, NEET and Class 8 to 10, grab our app now.
