In ΔPQR, PD ⊥ QR such that D lies on QR,

Given In A PQR, PD 1 QR, PQ = a, PR = b,QD = c and DR =d

To prove (a + b) (a-b) = (c + d)(c-d)

Proof In right angled ΔPDQ,

$P Q^{2}=P D^{2}+Q D^{2}$ [by Pythagoras theorem]

$\Rightarrow \quad a^{2}=P D^{2}+c^{2}$

$\Rightarrow \quad P D^{2}=a^{2}-c^{2}$ $\ldots$ (i)

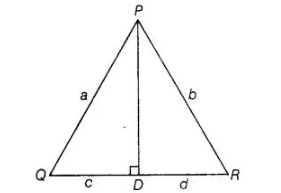
In right angled $\triangle P D R, \quad P R^{2}=P D^{2}+D R^{2} \quad$ [by Pythagoras theorem]

$\Rightarrow \quad b^{2}=P D^{2}+d^{2}$

$\Rightarrow \quad P D^{2}=b^{2}-d^{2}$ (ii)

From Eqs. (i) and (ii),

$a^{2}-c^{2}=b^{2}-d^{2}$

$\Rightarrow \quad a^{2}-b^{2}=c^{2}-d^{2}$

$\Rightarrow \quad(a-b)(a+b)=(c-d)(c+d)$

Hence proved.