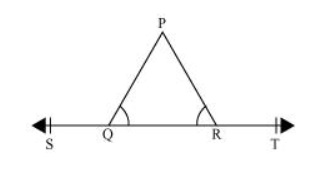
In the given figure, $\angle \mathrm{PQR}=\angle \mathrm{PRQ}$, then prove that $\angle \mathrm{PQS}=\angle \mathrm{PRT}$.

Solution:

In the given figure, ST is a straight line and ray QP stands on it.

$\therefore \angle \mathrm{PQS}+\angle \mathrm{PQR}=180^{\circ}$ (Linear Pair)

$\angle P Q R=180^{\circ}-\angle P Q S$(1)

$\angle \mathrm{PRT}+\angle \mathrm{PRQ}=180^{\circ}($ Linear Pair $)$

$\angle P R Q=180^{\circ}-\angle P R T(2)$

It is given that $\angle \mathrm{PQR}=\angle \mathrm{PRQ}$.

Equating equations (1) and (2), we obtain

$180^{\circ}-\angle \mathrm{PQS}=180^{\circ}-\angle \mathrm{PRT}$

$\angle \mathrm{PQS}=\angle \mathrm{PRT}$