Question:
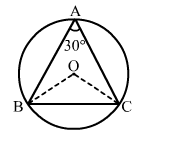
In the given figure, ∠BAC = 30°. Show that BC is equal to the radius of the circumcircle of ∆ABC whose centre is O.
Solution:
Join OB and OC.
∠BOC = 2∠BAC (As angle subtended by an arc of a circle at the centre is double the angle subtended by the arc at any point on the circumference)
= 2 × 30° [∵ ∠BAC = 30°]
= 60° ...(i)
Consider ΔBOC, we have:
OB = OC [Radii of a circle]
⇒ ∠OBC = ∠OCB ...(ii)
In ΔBOC, we have:
∠BOC + ∠OBC + ∠OCB = 180 (Angle sum property of a triangle)
⇒ 60° + ∠OCB + ∠OCB = 180° [From (i) and (ii)]
⇒ 2∠OCB = (180° - 60°) = 120°
⇒ ∠OCB = 60° ...(ii)
Thus we have:
∠OBC = ∠OCB = ∠BOC = 60°
Hence, ΔBOC is an equilateral triangle.
i.e., OB = OC = BC
∴ BC is the radius of the circumcircle.
