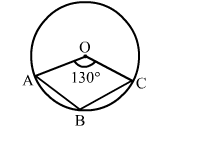
In the given figure, O is the centre of a circle and ∠AOC = 130°. Then, ∠ABC = ?

(c) 115°
Take a point D on the remaining part of the circumference.
Join AD and CD.

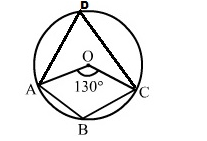
Then $\angle \mathrm{ADC}=\frac{1}{2} \angle \mathrm{AOC}=\left(\frac{1}{2} \times 130^{\circ}\right)=65^{\circ}$

In cyclic quadrilateral ABCD, we have:
∠ABC + ∠ADC = 180°     (Opposite angles of a cyclic quadrilateral)
⇒ ∠ABC + 65° = 180°
⇒ ∠ABC  = (180° - 65°) = 115°