In the given figure, the side $Q R$ of $\triangle P Q R$ is produced to a point $S$. If the bisectors of $\angle P Q R$ and $\angle P R S$ meet at point $T$, then prove that $\angle Q T R=$ $\frac{1}{2} \angle \mathrm{QPR}$

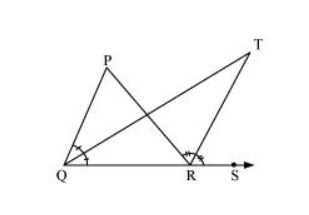
Solution:

In $\triangle Q T R, \angle T R S$ is an exterior angle.

$\therefore \angle \mathrm{QTR}+\angle \mathrm{TQR}=\angle \mathrm{TRS}$

$\angle Q T R=\angle T R S-\angle T Q R(1)$

For $\triangle P Q R, \angle P R S$ is an external angle.

$\therefore \angle \mathrm{QPR}+\angle \mathrm{PQR}=\angle \mathrm{PRS}$

$\angle Q P R+2 \angle T Q R=2 \angle T R S($ As $Q T$ and $R T$ are angle bisectors $)$

$\angle Q P R=2(\angle T R S-\angle T Q R)$

$\angle Q P R=2 \angle Q T R$ [By using equation (1)]

$\angle Q T R=\frac{1}{2} \angle Q P R$