Question:
In three line segments OA, OB and OC points L, M, N respectively are so chosen that LM || AB and MN || BC but neither of L, M, N nor of A, B, C are collinear. Show that LN || AC.
Solution:
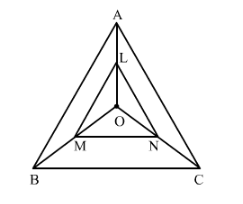
In ∆OAB, since LM∥AB, thenOLLA = OMMB By BPT ........1In ∆OBC, since MN∥BC, then OMMB = ONNC By BPT ⇒ ONNC = OMMB .........2from 1 and 2, we get OLLA = ONNC
Click here to get exam-ready with eSaral
For making your preparation journey smoother of JEE, NEET and Class 8 to 10, grab our app now.
