Question:
In $\triangle \mathrm{ABC}$, the lengths of sides $\mathrm{AC}$ and $\mathrm{AB}$ are $12 \mathrm{~cm}$ and $5 \mathrm{~cm}$, respectively. If the area of $\triangle \mathrm{ABC}$ is $30 \mathrm{~cm}^{2}$ and $\mathrm{R}$ and $\mathrm{r}$ are respectively the radii of circumcircle and incircle of $\triangle \mathrm{ABC}$, then the value of $2 R+r$ (in $\mathrm{cm}$ ) is equal to_____.
Solution:
$\Delta=\frac{1}{2} .5 .12 . \sin \mathrm{A}=30$
$\sin A=1$
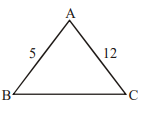
$\mathrm{A}=90^{\circ} \Rightarrow \mathrm{BC}=13$
$\mathrm{BC}=2 \mathrm{R}=13$
$r=\frac{\Delta}{S}=\frac{30}{15}=2$
$2 \mathrm{R}+\mathrm{r}=15$
Click here to get exam-ready with eSaral
For making your preparation journey smoother of JEE, NEET and Class 8 to 10, grab our app now.
