Question:
Let f: X → Y be an invertible function. Show that f has unique inverse.
(Hint: suppose g1 and g2 are two inverses of f. Then for all y ∈ Y,
fog1(y) = IY(y) = fog2(y). Use one-one ness of f).
Solution:
Let f: X → Y be an invertible function.
Also, suppose $f$ has two inverses (say $g_{1}$ and $g_{2}$ ).
Then, for all $y \in Y$, we have:
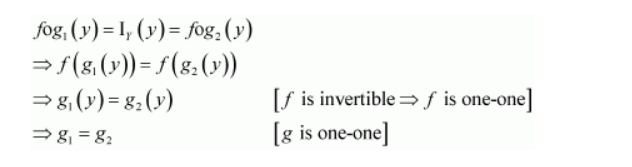
Hence, f has a unique inverse.
Click here to get exam-ready with eSaral
For making your preparation journey smoother of JEE, NEET and Class 8 to 10, grab our app now.
