Let $S$ and $S^{\prime}$ be the foci of the ellipse and $B$ be any one of the extremities of its minor axis. If $\Delta \mathrm{S} ' \mathrm{BS}$ is a right angled triangle with right angle at $B$ and area $\left(\Delta S^{\prime} B S\right)=8$ sq. units, then the length of a latus rectum of the ellipse is :
Correct Option: , 3
$\mathrm{m}_{\mathrm{SB}} \cdot \mathrm{m}_{\mathrm{SB}}=-1$
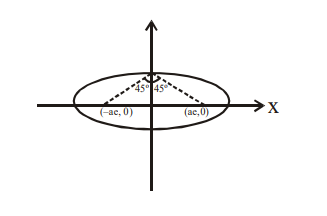
$b^{2}=a^{2} e^{2} \quad \ldots .$ (i)
$\frac{1}{2} S^{\prime} B \cdot S B=8$
S'B. $\mathrm{SB}=16$
$a^{2} e^{2}+b^{2}=16 \ldots . .$ (ii)
$b^{2}=a^{2}\left(1-e^{2}\right) \ldots .$ (iii)
using (i),(ii), (iii) $a=4$
$b=2 \sqrt{2}$
$e=\frac{1}{\sqrt{2}}$
$\therefore \ell($ L.R $)=\frac{2 b^{2}}{\mathrm{a}}=4 \quad$ Ans.3
Click here to get exam-ready with eSaral
For making your preparation journey smoother of JEE, NEET and Class 8 to 10, grab our app now.
