Let the curve $y=y(x)$ be the solution of the differential equation, $\frac{\mathrm{dy}}{\mathrm{dx}}=2(\mathrm{x}+1)$. If the
numerical value of area bounded by the curve
$y=y(x)$ and $x$-axis is $\frac{4 \sqrt{8}}{3}$, then the value of $\mathrm{y}(1)$ is equal to______.
$\frac{\mathrm{dy}}{\mathrm{dx}}=2(\mathrm{x}+1)$
$\Rightarrow \int d y=\int 2(x+1) d x$
$\Rightarrow y(x)=x^{2}+2 x+C$
Area $=\frac{4 \sqrt{8}}{3}$
$-1+\sqrt{1-C}$
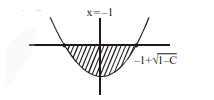
$\Rightarrow \quad 2 \int_{-1}^{-1+\sqrt{1-C}}\left(-(x+1)^{2}-C+1\right) d x=\frac{4 \sqrt{8}}{3}$
$\Rightarrow 2\left[-\frac{(x+1)^{3}}{3}-C x+x\right]_{-1}^{-1+\sqrt{1-c}}=\frac{4 \sqrt{8}}{3}$
$\Rightarrow \quad-(\sqrt{1-\mathrm{C}})^{3}+3 \mathrm{c}-3 \mathrm{C} \sqrt{1-\mathrm{C}}$
$-3+3 \sqrt{1-\mathrm{C}}-3 \mathrm{C}+3=2 \sqrt{8}$
$\Rightarrow C=-1$
$\Rightarrow f(x)=x^{2}+2 x-1, \quad f(1)=2$