Match the AP’s given in column

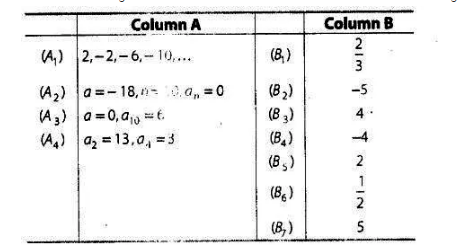
A, $2,-2,-6,-10, \ldots$

Here, common difference. $d=-2-2=-4$

$A_{2} . \because$ $a_{t,} a+(n-1) d$

$\Rightarrow$ $0=-18+(10-1) d$

$18=9 d$

$\therefore$ Common difference, $d=2$

$A_{3} \cdot \because \quad a_{10}=6$

$\Rightarrow \quad a+(10-1) d=6$

$\Rightarrow \quad 0+9 d=6$             $[\because a=0$ (given) $]$

$9 d=6 \Rightarrow d=\frac{2}{3}$ 

$A_{4} \because \quad a_{2}=13$

$\Rightarrow \quad a+(2-1) d=13 \quad\left[\because a_{n}=a+(n-1) d\right]$

$\Rightarrow \quad a+d=13 \quad \ldots(i)$

and $\quad a_{4}=3 \Rightarrow a+(4-1) d=3$

$\therefore \quad a+3 d=3$ ....(ii)

On subtracting Eq. (i) from Eq. (ii), we get

$2 d=-10$

$\Rightarrow \quad d=-5$

$\therefore \quad\left(A_{1}\right) \rightarrow B_{4},\left(A_{2}\right) \rightarrow B_{51}\left(A_{3}\right) \rightarrow B_{1}$ and $\left(A_{4}\right) \rightarrow B_{2}$