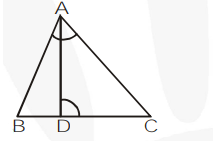
$\mathrm{D}$ is a point on the side $\mathrm{BC}$ of a triangle $\mathrm{ABC}$ such that $\angle \mathrm{ADC}=\angle \mathrm{BAC}$.
Question.
$\mathrm{D}$ is a point on the side $\mathrm{BC}$ of a triangle $\mathrm{ABC}$ such that $\angle \mathrm{ADC}=\angle \mathrm{BAC}$. Show that $\mathrm{CA}^{2}=$ CB. CD.
$\mathrm{D}$ is a point on the side $\mathrm{BC}$ of a triangle $\mathrm{ABC}$ such that $\angle \mathrm{ADC}=\angle \mathrm{BAC}$. Show that $\mathrm{CA}^{2}=$ CB. CD.
Solution:
For $\triangle \mathrm{ABC}$ and $\triangle \mathrm{DAC}$, We have
$\angle \mathrm{BAC}=\angle \mathrm{ADC} \quad($ Given $)$
and $\angle \mathrm{ACB}=\angle \mathrm{DCA} \quad($ Each $=\angle \mathrm{C})$
$\Rightarrow \Delta \mathrm{ABC} \sim \Delta \mathrm{DAC} \quad$ (AA similarity)
$\Rightarrow \frac{A C}{D C}=\frac{C B}{C A}$
$\Rightarrow \frac{C A}{C D}=\frac{C B}{C A}$
$\Rightarrow \mathrm{CA} \times \mathrm{CA}=\mathrm{CB} \times \mathrm{CD}$
$\Rightarrow \mathrm{CA}^{2}=\mathrm{CB} \times \mathrm{CD}$
For $\triangle \mathrm{ABC}$ and $\triangle \mathrm{DAC}$, We have
$\angle \mathrm{BAC}=\angle \mathrm{ADC} \quad($ Given $)$
and $\angle \mathrm{ACB}=\angle \mathrm{DCA} \quad($ Each $=\angle \mathrm{C})$
$\Rightarrow \Delta \mathrm{ABC} \sim \Delta \mathrm{DAC} \quad$ (AA similarity)
$\Rightarrow \frac{A C}{D C}=\frac{C B}{C A}$
$\Rightarrow \frac{C A}{C D}=\frac{C B}{C A}$
$\Rightarrow \mathrm{CA} \times \mathrm{CA}=\mathrm{CB} \times \mathrm{CD}$
$\Rightarrow \mathrm{CA}^{2}=\mathrm{CB} \times \mathrm{CD}$