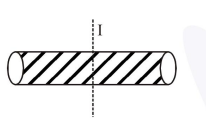
Moment of inertia of a cylinder of mass M, length $\mathrm{L}$ and radius $\mathrm{R}$ about an axis passing through its centre and perpendicular to the axis
of the cylinder is $\mathrm{I}=\mathrm{M}\left(\frac{\mathrm{R}^{2}}{4}+\frac{\mathrm{L}^{2}}{12}\right)$. If such a
cylinder is to be made for a given mass of material, the ratio $\mathrm{L} / \mathrm{R}$ for it to have minimum possible I is :-
Correct Option: , 3
$\mathrm{I}=\mathrm{M}\left(\frac{\mathrm{R}^{2}}{4}+\frac{\mathrm{L}^{2}}{12}\right)$..........(1)
as mass is constant $\Rightarrow \mathrm{m}=\rho \mathrm{V}=$ constant
$\mathrm{V}=$ constant
$\pi^{2} \mathrm{R} l=$ constant $\Rightarrow \mathrm{R}^{2} \mathrm{~L}=$ constant
$2 \mathrm{RL}+\mathrm{R}^{2} \frac{\mathrm{dL}}{\mathrm{dR}}=0$.......(2)
From equation (1)
$\frac{\mathrm{dI}}{\mathrm{dR}}=\mathrm{M}\left(\frac{2 \mathrm{R}}{4}+\frac{2 \mathrm{~L}}{12} \times \frac{\mathrm{dL}}{\mathrm{dr}}\right)=0$
$\frac{\mathrm{R}}{2}+\frac{\mathrm{L}}{6} \frac{\mathrm{dL}}{\mathrm{dR}}=0$
Substituting value of $\frac{\mathrm{dL}}{\mathrm{dR}}$ from eqution (2)
$\frac{R}{2}+\frac{L}{6}\left(\frac{-2 L}{R}\right)=0$
$\frac{\mathrm{R}}{2}=\frac{\mathrm{L}^{2}}{3 \mathrm{R}} \Rightarrow \frac{\mathrm{L}}{\mathrm{R}}=\sqrt{\frac{3}{2}}$
