Obtain the equivalent capacitance of the network in Fig. 2.35. For a 300 V supply, determine the charge and voltage across each capacitor.

Capacitance of capacitor C1 is 100 pF.
Capacitance of capacitor C2 is 200 pF.
Capacitance of capacitor C3 is 200 pF.
Capacitance of capacitor C4 is 100 pF.
Capacitors $C_{2}$ and $C_{3}$ are connected in series. Let their equivalent capacitance be $C^{\prime}$.
$\therefore \frac{1}{C^{\prime}}=\frac{1}{200}+\frac{1}{200}=\frac{2}{200}$
$C^{\prime}=100 \mathrm{pF}$
Capacitors $C_{1}$ and $C^{\prime}$ are in parallel. Let their equivalent capacitance be $C^{\prime \prime}$.
$\therefore C^{\prime \prime}=C^{\prime}+\mathrm{C}_{1}$
$=100+100=200 \mathrm{pF}$
$C^{\prime \prime}$ and $C_{4}$ are connected in series. Let their equivalent capacitance be $C$.
$\therefore \frac{1}{C}=\frac{1}{C^{\prime \prime}}+\frac{1}{C_{4}}$
$=\frac{1}{200}+\frac{1}{100}=\frac{2+1}{200}$
$C=\frac{200}{3} \mathrm{pF}$
Hence, the equivalent capacitance of the circuit is $\frac{200}{3} \mathrm{pF}$.
Potential difference across $C^{\prime \prime}=V^{\prime \prime}$
Potential difference across C4 = V4
$\therefore V^{\prime \prime}+V_{4}=V=300 \mathrm{~V}$
Charge on $C_{4}$ is given by,
$Q_{4}=C V$
$=\frac{200}{3} \times 10^{-12} \times 300$
$=2 \times 10^{-8} \mathrm{C}$
$\therefore V_{4}=\frac{Q_{4}}{C_{4}}$
$=\frac{2 \times 10^{-8}}{100 \times 10^{-12}}=200 \mathrm{~V}$
$\therefore$ Voltage across $C_{1}$ is given by,
$V_{1}=V-V_{4}$
$=300-200=100 \mathrm{~V}$
Hence, potential difference, $V_{1}$, across $C_{1}$ is $100 \mathrm{~V}$.
Charge on $C_{1}$ is given by,
$Q_{1}=C_{1} V_{1}$
$=100 \times 10^{-12} \times 100$
$=10^{-8} \mathrm{C}$
$C_{2}$ and $C_{3}$ having same capacitances have a potential difference of $100 \mathrm{~V}$ together. Since $C_{2}$ and $C_{3}$ are in series, the potential difference across $C_{2}$ and $C_{3}$ is given by,
$V_{2}=V_{3}=50 \mathrm{~V}$
Therefore, charge on $C_{2}$ is given by,
$Q_{2}=C_{2} V_{2}$
$=200 \times 10^{-12} \times 50$
$=10^{-8} \mathrm{C}$
And charge on $C_{3}$ is given by,
$Q_{3}=C_{3} V_{3}$
$=200 \times 10^{-12} \times 50$
$=10^{-8} \mathrm{C}$
Hence, the equivalent capacitance of the given circuit is $\frac{200}{3} \mathrm{pF}$ with,
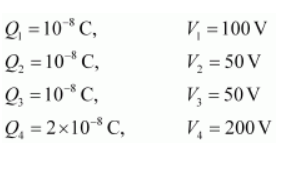
Click here to get exam-ready with eSaral
For making your preparation journey smoother of JEE, NEET and Class 8 to 10, grab our app now.
