P and Q are respectively the mid-points of sides AB and BC of a triangle

Solution:

Take a point $S$ on $A C$ such that $S$ is the mid-point of $A C$.

Extend $P Q$ to $T$ such that $P Q=Q T$.

Join TC, QS, PS, and AQ.



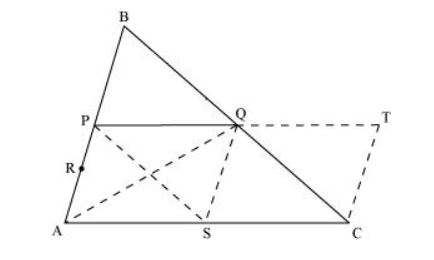
In ΔABC, P and Q are the mid-points of AB and BC respectively. Hence, by using mid-point theorem, we obtain

$P Q \| A C$ and $P Q=\frac{1}{2} A C$

$\Rightarrow P Q \| A S$ and $P Q=A S(A s S$ is the mid-point of $A C)$

$\therefore$ PQSA is a parallelogram. We know that diagonals of a parallelogram bisect it into equal areas of triangles.

$\therefore \operatorname{ar}(\Delta \mathrm{PAS})=\operatorname{ar}(\Delta \mathrm{SQP})=\operatorname{ar}(\Delta \mathrm{PAQ})=\operatorname{ar}(\Delta \mathrm{SQA})$

Similarly, it can also be proved that quadrilaterals PSCQ, QSCT, and PSQB are also parallelograms and therefore,

ar (ΔPSQ) = ar (ΔCQS) (For parallelogram PSCQ)

ar (ΔQSC) = ar (ΔCTQ) (For parallelogram QSCT)

ar (ΔPSQ) = ar (ΔQBP) (For parallelogram PSQB)

Thus,

$\operatorname{ar}(\Delta \mathrm{PAS})=\operatorname{ar}(\Delta \mathrm{SQP})=\operatorname{ar}(\Delta \mathrm{PAQ})=\operatorname{ar}(\Delta \mathrm{SQA})=\operatorname{ar}(\Delta \mathrm{QSC})=\operatorname{ar}(\Delta \mathrm{CTQ})=\operatorname{ar}(\Delta \mathrm{QBP}) \ldots(1)$

Also, $\operatorname{ar}(\triangle \mathrm{ABC})=\operatorname{ar}(\triangle \mathrm{PBQ})+\operatorname{ar}(\triangle \mathrm{PAS})+\operatorname{ar}(\triangle \mathrm{PQS})+\operatorname{ar}(\triangle \mathrm{QSC})$

$\operatorname{ar}(\Delta \mathrm{ABC})=\operatorname{ar}(\Delta \mathrm{PBQ})+\operatorname{ar}(\Delta \mathrm{PBQ})+\operatorname{ar}(\Delta \mathrm{PBQ})+\operatorname{ar}(\Delta \mathrm{PBQ})$

$=\operatorname{ar}(\Delta \mathrm{PBQ})+\operatorname{ar}(\Delta \mathrm{PBQ})+\operatorname{ar}(\Delta \mathrm{PBQ})+\operatorname{ar}(\Delta \mathrm{PBQ})$

$=4 \operatorname{ar}(\Delta P B Q)$

$\Rightarrow \operatorname{ar}(\Delta \mathrm{PBQ})=\frac{1}{4} \operatorname{ar}(\triangle \mathrm{ABC}) \ldots(2)$

(i)Join point P to C.



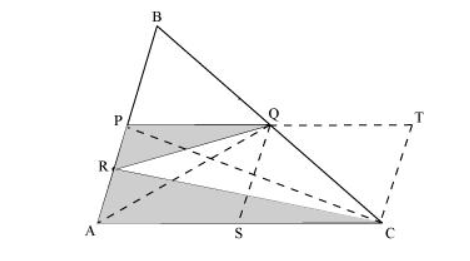
In $\triangle \mathrm{PAQ}, \mathrm{QR}$ is the median.

$\therefore \operatorname{ar}(\Delta \mathrm{PRQ})=\frac{1}{2} \operatorname{ar}(\Delta \mathrm{PAQ})=\frac{1}{2} \times \frac{1}{4} \operatorname{ar}(\Delta \mathrm{ABC})=\frac{1}{8} \operatorname{ar}(\Delta \mathrm{ABC}) \ldots$(3)

In ΔABC, P and Q are the mid-points of AB and BC respectively. Hence, by using mid-point theorem, we obtain

$P Q=\frac{1}{2} A C$

$\mathrm{AC}=2 \mathrm{PQ} \Rightarrow \mathrm{AC}=\mathrm{PT}$

Also, $P Q\|A C \Rightarrow P T\| A C$

Hence, PACT is a parallelogram.

$\operatorname{ar}(\mathrm{PACT})=\operatorname{ar}(\mathrm{PACQ})+\operatorname{ar}(\Delta \mathrm{QTC})$

$=\operatorname{ar}(\mathrm{PACQ})+\operatorname{ar}(\Delta \mathrm{PBQ}[\cup \operatorname{sing}$ equation $(1)]$

$\therefore \operatorname{ar}(\mathrm{PACT})=\operatorname{ar}(\triangle \mathrm{ABC}) \ldots$(4)

$\operatorname{ar}(\Delta \mathrm{ARC})=\frac{1}{2} \operatorname{ar}(\Delta \mathrm{PAC})$

(CR is the median of $\triangle \mathrm{PAC}$ )

$=\frac{1}{2} \times \frac{1}{2} \operatorname{ar}(\mathrm{PACT})(\mathrm{PC}$ is the diagonal of parallelogram $\mathrm{PACT})$'

$=\frac{1}{4} \operatorname{ar}(\Delta \mathrm{PACT})=\frac{1}{4} \operatorname{ar}(\Delta \mathrm{ABC})$

$\Rightarrow \frac{1}{2} \operatorname{ar}(\Delta \mathrm{ARC})=\frac{1}{8} \operatorname{ar}(\Delta \mathrm{ABC})$

$\Rightarrow \frac{1}{2} \operatorname{ar}(\Delta \mathrm{ARC})=\operatorname{ar}(\Delta \mathrm{PRQ})$ [Using equation (3)]..(5)



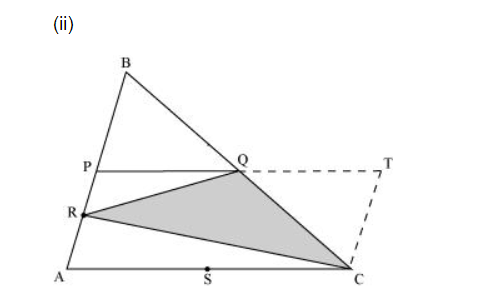
$\operatorname{ar}(\mathrm{PACT})=\operatorname{ar}(\Delta \mathrm{PRQ})+\operatorname{ar}(\Delta \mathrm{ARC})+\operatorname{ar}(\Delta \mathrm{QTC})+\operatorname{ar}(\Delta \mathrm{RQC})$

Putting the values from equations (1), (2), (3), (4), and (5), we obtain

$\operatorname{ar}(\Delta \mathrm{ABC})=\frac{1}{8} \operatorname{ar}(\Delta \mathrm{ABC})+\frac{1}{4} \operatorname{ar}(\Delta \mathrm{ABC})+\frac{1}{4} \operatorname{ar}(\Delta \mathrm{ABC})+\operatorname{ar}(\Delta \mathrm{RQC})$

$\operatorname{ar}(\triangle \mathrm{ABC})=\frac{5}{8} \operatorname{ar}(\triangle \mathrm{ABC})+\operatorname{ar}(\triangle \mathrm{RQC})$

$\operatorname{ar}(\Delta \mathrm{RQC})=\left(1-\frac{5}{8}\right) \operatorname{ar}(\Delta \mathrm{ABC})$

$\operatorname{ar}(\Delta R Q C)=\frac{3}{8} \operatorname{ar}(\Delta A B C)$

(iii) In parallelogram PACT,

$\operatorname{ar}(\Delta \mathrm{ARC})=\frac{1}{2} \operatorname{ar}(\Delta \mathrm{PAC})$

(CR is the median of $\triangle \mathrm{PAC}$ )

$=\frac{1}{2} \times \frac{1}{2} \operatorname{ar}(\mathrm{PACT})(\mathrm{PC}$ is the diagonal of parallelogram $\mathrm{PACT})$

$=\frac{1}{4} \operatorname{ar}(\Delta \mathrm{PACT})$

$=\frac{1}{4} \operatorname{ar}(\Delta \mathrm{ABC})$

$=\operatorname{ar}(\Delta \mathrm{PBQ})$