Proton with kinetic energy of $1 \mathrm{MeV}$ moves from south to north. It gets an acceleration of $10^{12} \mathrm{~m} / \mathrm{s}^{2}$ by an applied magnetic field (west to east). The value of magnetic field: (Rest mass of proton is $1.6 \times 10^{-27} \mathrm{~kg}$ )
Correct Option: 1
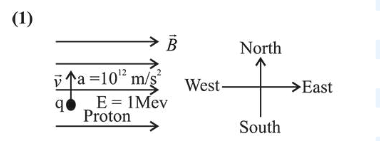
As we know, magnetic force $F=q v B=m a$
$\therefore \vec{a}=\left(\frac{q v B}{m}\right)$ perpendicular to velocity.
$\therefore$ Also $v=\sqrt{\frac{2 K E}{m}}=\sqrt{\frac{2 \times e \times 10^{6}}{m}}$
$\therefore a=\frac{q v B}{m}=\frac{e B}{m} \sqrt{\frac{2 \times e \times 10^{6}}{m}}$
$\therefore B \simeq \frac{1}{\sqrt{2}} \times 10^{-3} T=0.71 \mathrm{mT}($ approx $)$
$\therefore 10^{12}=\left(\frac{1.6 \times 10^{-19}}{1.67 \times 10^{-27}}\right)^{\frac{3}{2}} \cdot \sqrt{2} \times 10^{3} B$
