Prove that:

Let $A=\left[a_{i j}\right]$ be a square matrix of order $n \times n$.

If $C_{i j}$ is a cofactor of $a_{i j}$ in $A$, then adj $A=\left[C_{\mathrm{i} j}\right]^{T}=\left[C_{\mathrm{ji}}\right] .$ Also, it is a matrix of order $n \times n$.

Because $A$ and adj $A$ are matrices of order $n \times n, A \times(\operatorname{adj} A)$ exists and is of order $n \times n$.

$\Rightarrow\{A \times(\operatorname{adj} A)\}_{\mathrm{ij}}=\sum_{r=1}^{n} A_{i r}(\operatorname{adj} A)_{r j}$

$=\sum_{r=1}^{n} a_{i r} C_{r j}=\left\{\begin{array}{lr}|A| & \text { if } i=j \\ 0 & \text { otherwise }\end{array}\right.$

Thus, each diagonal element of $\mathrm{A} \times(\operatorname{adj} \mathrm{A})$ is $|\mathrm{A}| .$ Also, the non - diagonal elements are zero.

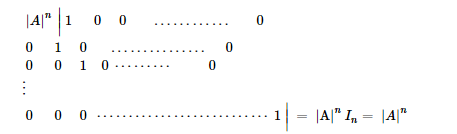
$\Rightarrow|A \times(\operatorname{adj} A)|=|A|^{n}$

Hence proved.