Prove that

Given: $\operatorname{cosec} \theta=\frac{5}{3}$

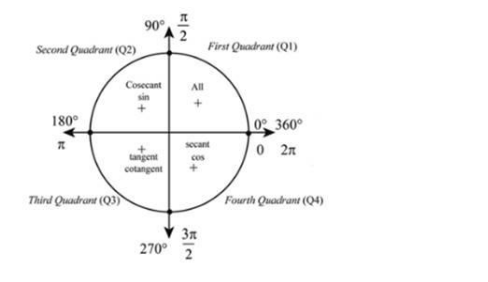
Since, θ is in IInd Quadrant. So, cos and tan will be negative but sin will be positive.

Now, we know that

$\sin \theta=\frac{1}{\operatorname{cosec} \theta}$

Putting the values, we get

$\sin \theta=\frac{1}{\frac{5}{3}}$

$\sin \theta=\frac{3}{5}$ …(i)

We know that,

$\sin ^{2} \theta+\cos ^{2} \theta=1$

Putting the values, we get

$\left(\frac{3}{5}\right)^{2}+\cos ^{2} \theta=1$ [from (i)]

$\Rightarrow \frac{9}{25}+\cos ^{2} \theta=1$

$\Rightarrow \cos ^{2} \theta=1-\frac{9}{25}$

$\Rightarrow \cos ^{2} \theta=\frac{25-9}{25}$

$\Rightarrow \cos ^{2} \theta=\frac{16}{25}$

$\Rightarrow \cos \theta=\sqrt{\frac{16}{25}}$

$\Rightarrow \cos \theta=\pm \frac{4}{5}$

Since, $\theta$ in IInd quadrant and $\cos \theta$ is negative in II $^{\text {nd }}$ quadrant

$\therefore \cos \theta=-\frac{4}{5}$

Now,

$\tan \theta=\frac{\sin \theta}{\cos \theta}$

Putting the values, we get

$\tan \theta=\frac{\frac{3}{5}}{-\frac{4}{5}}$

$=\frac{3}{5} \times\left(-\frac{5}{4}\right)$

$=-\frac{3}{4}$

Now,

$\sec \theta=\frac{1}{\cos \theta}$

Putting the values, we get

$\sec \theta=\frac{1}{-\frac{4}{5}}$

$=-\frac{5}{4}$

Now,

$\cot \theta=\frac{1}{\tan \theta}$

Putting the values, we get

$\cot \theta=\frac{1}{-\frac{3}{4}}$

$=-\frac{4}{3}$

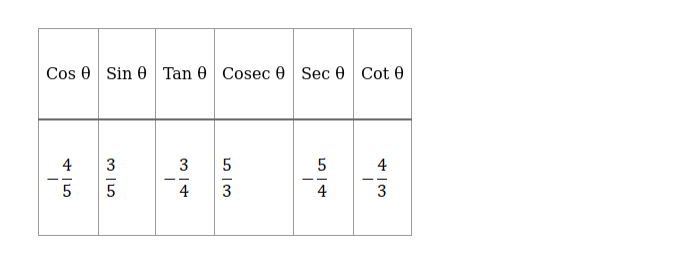
Hence, the values of other trigonometric Functions are