Question:
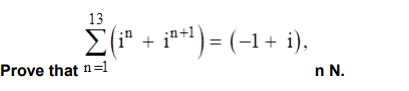
Solution:
L.H.S $=\sum_{n=1}^{13}\left(i^{n}+i^{n+1}\right)$
$=i^{1}+i^{2}+i^{3}+i^{4}+i^{5}+i^{6}+\ldots . .+i^{13}+i^{14}$
Since $i^{4^{4 n}}=1$
$\Rightarrow i^{4 n+1}=i$
$\Rightarrow i^{4 n+2}=-1$
$\Rightarrow i^{4 n+3}=-1$
$=i-1-i+1+i-1 \ldots \ldots+i-1$
As, all terms will get cancel out consecutively except the first two terms. So that will get remained will be the answer.
Activate Windows
$=\mathrm{i}-1$
L.H.S = R.H.S
Hence proved.