Prove that the opposite sides of a quadrilateral circumscribing a circle

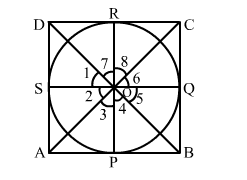
Given, a quadrilateral ABCD circumscribes a circle with centre O.

To prove: $\angle A O B+\angle C O D=180^{\circ}$

and $\angle A O D+\angle B O C=180^{\circ}$

Join $O P, O Q, O R$ and $O S$.

We know that the tangents drawn from an external point of a circle

subtend equal angles at the centre.

$\therefore \angle 1=\angle 7, \angle 2=\angle 3, \angle 4=\angle 5$ and $\angle 6=\angle 8$

and $\angle 1+\angle 2+\angle 3+\angle 4+\angle 5+\angle 6+\angle 7+\angle 8=360^{\circ} \quad$ [angles at a point]

$\Rightarrow(\angle 1+\angle 7)+(\angle 3+\angle 2)+(\angle 4+\angle 5)+(\angle 6+\angle 8)=360^{0}$

$2 \angle 1+2 \angle 2+2 \angle 6+2 \angle 5=360^{\circ}$

$\Rightarrow \angle 1+\angle 2+\angle 5+\angle 6=180^{\circ}$

$\Rightarrow \angle A O B+\angle C O D=180^{\circ}$ and $\angle A O D+\angle B O C=180^{\circ}$