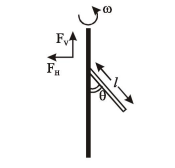
A uniform rod of length ' $l$ ' is pivoted at one of its ends on a vertical shaft of negligible radius. When the shaft rotates at angular speed $\omega$ the rod makes an angle $\theta$ with it (see figure). To find $\theta$ equate the rate of change of angular momentum (direction going into the paper)
$\frac{m \ell^{2}}{12} \omega^{2} \sin \theta \cos \theta$ about the centre of mass
(CM) to the torque provided by the horizontal and vertical forces $\mathrm{F}_{\mathrm{H}}$ and $\mathrm{F}_{\mathrm{V}}$ about the $\mathrm{CM}$. The value of $\theta$ is then such that:
Correct Option: , 2
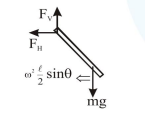
$\mathrm{F}_{\mathrm{V}}=\mathrm{mg}$
$\mathrm{F}_{\mathrm{H}}=\mathrm{m} \omega^{2} \frac{\ell}{2} \sin \theta$
$\mathrm{mg} \frac{\ell}{2} \sin \theta-\mathrm{m} \omega^{2} \frac{\ell}{2} \sin \theta \frac{\ell}{2} \cos \theta=\frac{\mathrm{m} \ell^{2}}{12} \omega^{2} \sin \theta \cos \theta$
$\cos \theta=\frac{3}{2} \frac{g}{\omega^{2} \ell}$ ..........(ii)
Click here to get exam-ready with eSaral
For making your preparation journey smoother of JEE, NEET and Class 8 to 10, grab our app now.
