Question.
Show that the area of the triangle contained between the vectors $\mathbf{a}$ and $\mathbf{b}$ is one half of the magnitude of $\mathbf{a} \times \mathbf{b}$.
Show that the area of the triangle contained between the vectors $\mathbf{a}$ and $\mathbf{b}$ is one half of the magnitude of $\mathbf{a} \times \mathbf{b}$.
solution:
Consider two vectors $\overrightarrow{\mathrm{OK}}=|\vec{a}|$ and $\overrightarrow{\mathrm{OM}}=|\vec{b}|$, inclined at an angle $\theta$, as shown in the following figure.
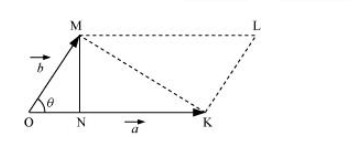
In $\Delta \mathrm{OMN}$, we can write the relation:
$\sin \theta=\frac{\mathrm{MN}}{\mathrm{OM}}=\frac{\mathrm{MN}}{|\vec{b}|}$
$\mathrm{MN}=|\vec{b}| \sin \theta$
$|\vec{a} \times \vec{a}|=|\vec{a}||\vec{b}| \sin \theta$
$=\mathrm{OK} \cdot \mathrm{MN} \times \frac{2}{2}$
$=2 \times$ Area of $\Delta$ OMK
$=2 \times$ Area of $\Delta \mathrm{OMK}$
$\therefore$ Area of $\triangle \mathrm{OMK}=\frac{1}{2}|\vec{a} \times \vec{b}|$
Consider two vectors $\overrightarrow{\mathrm{OK}}=|\vec{a}|$ and $\overrightarrow{\mathrm{OM}}=|\vec{b}|$, inclined at an angle $\theta$, as shown in the following figure.
In $\Delta \mathrm{OMN}$, we can write the relation:
$\sin \theta=\frac{\mathrm{MN}}{\mathrm{OM}}=\frac{\mathrm{MN}}{|\vec{b}|}$
$\mathrm{MN}=|\vec{b}| \sin \theta$
$|\vec{a} \times \vec{a}|=|\vec{a}||\vec{b}| \sin \theta$
$=\mathrm{OK} \cdot \mathrm{MN} \times \frac{2}{2}$
$=2 \times$ Area of $\Delta$ OMK
$=2 \times$ Area of $\Delta \mathrm{OMK}$
$\therefore$ Area of $\triangle \mathrm{OMK}=\frac{1}{2}|\vec{a} \times \vec{b}|$