Solve the equation

Solution:

Given equation, cos (tan-1 x) = sin (cot-1 3/4)

Taking L.H.S,

We have, $\cos \left(\tan ^{-1} x\right)=\sin \left(\cot ^{-1} \frac{3}{4}\right)$

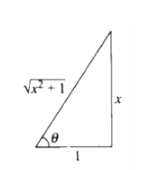
L.H.S. $=\cos \left(\tan ^{-1} x\right)$

$=\cos \left(\cos ^{-1} \frac{1}{\sqrt{x^{2}+1}}\right)$

$=\frac{1}{\sqrt{x^{2}+1}}$ $\left(\because \cos \left(\cos ^{-1} x\right)=x, x \in[-1,1]\right)$

R.H.S. $=\sin \left(\cot ^{-1} \frac{3}{4}\right)$

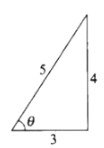
$=\sin \left(\sin ^{-1} \frac{4}{5}\right)$

$=\frac{4}{5}$     $\left(\because \sin \left(\sin ^{-1} x\right)=x, x \in[-1,1]\right)$

Hence, on equating the L.H.S and R.H.S,we get $\frac{1}{\sqrt{x^{2}+1}}=\frac{4}{5}$

On squaring on both sides,

16(x2 + 1) = 25

16x2 + 16 = 25

16x2 = 9

x2 = 9/16

Therefore, x = ± 3/4