A block of mass $200 \mathrm{~g}$ is suspended through a vertical spring. The spring is stretched by $1.0 \mathrm{~cm}$ when the block is in equilibrium. A particle of mass $120 \mathrm{~g}$ is dropped on the block from a height of $45 \mathrm{~cm}$. The particle sticks to the block after the impact. Find the maximum extension of the spring. Take $g=10 \mathrm{~m} / \mathrm{s}^{2}$.
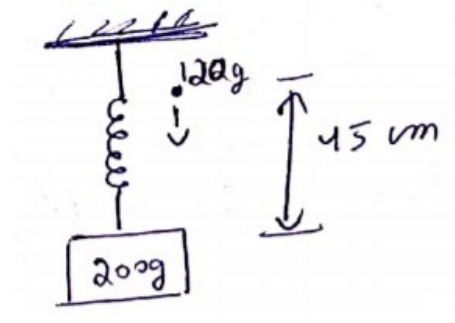
At equilibrium $k x_{0}=200 \mathrm{~g}$
(before $120 \mathrm{~g}$ falls) $k=\frac{0.200 \times 10}{0.01}=200 \mathrm{~N} / \mathrm{m}$
$v=\sqrt{2 g h}=\sqrt{2 \times 10 \times 0.45}=3 \mathrm{~m} / \mathrm{s}$.
Use C.O.L.M,
$(0.12) .3=(0.12+0.2) \mathrm{V}$
$V=\frac{0.12 \times 3}{0.32}=\frac{9}{8} \mathrm{~m} / \mathrm{s}$
If spring is stretched extra by $\Delta x$ According to conservation of energy,
$\frac{-1}{2}(0.12+0.2) \times\left(\frac{9}{8}\right)^{2}$
$=(0.12+0.2) \times 10 \times(\Delta x)-\frac{1}{2}(0.2)\left[(2+0.01)^{2}-(0.01)^{2}\right]$
$\Delta x=0.045=4.5 \mathrm{~cm}$
