If $f(x)=\frac{k x}{x+1}$, where $x \neq-1$ and $f\{f(x)\}=x$ for $x \neq-1$ then find the value of k.
Given. $f(x)=\frac{k x}{x+1}, x \neq-1$
$F(f(x))=f\left(\frac{k x}{(x+1)}\right.$
$=\frac{k \frac{k x}{x+1}}{\frac{k x}{x+1}+1}$
$=\frac{k^{2} x}{k x+x+1}$
Given that f(f(x)) = x
$\mathrm{x}=\frac{k^{2} \mathrm{x}}{k x+x+1}$
Dividing both sides by x
$1=\frac{k^{2}}{k x+x+1}$
$\mathrm{kx}+\mathrm{x}+1=k^{2}$
$1^{k^{2}}-k x-(x+1)=0$
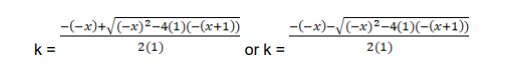
$\mathrm{k}=\frac{\frac{x+\sqrt{x^{2}+4 x+4}}{2}}{2}$ or $\mathrm{k}=\frac{x-\sqrt{x^{2}+4 x+4}}{2}$
$\mathrm{k}=\frac{\frac{x+x+2}{2}}{2}$ or $\mathrm{k}=\frac{x-x-2}{2}$
$k=x+1$ or $k=-1$
As value of x is variable we take k = -1.
Therefore, k= -1
Click here to get exam-ready with eSaral
For making your preparation journey smoother of JEE, NEET and Class 8 to 10, grab our app now.
