Question:
In a triangle $\mathrm{ABC}$, if $|\overrightarrow{\mathrm{BC}}|=8,|\overrightarrow{\mathrm{CA}}|=7$,
$|\overrightarrow{\mathrm{AB}}|=10$, then the projection of the vector $\overrightarrow{\mathrm{AB}}$
on $\overrightarrow{\mathrm{AC}}$ is equal to :
Correct Option: , 2
Solution:
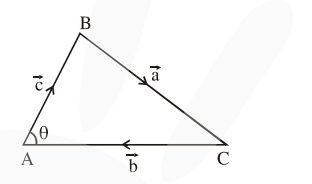
$|\vec{a}|=8,|\vec{b}|=7,|\vec{c}|=10$
$\cos \theta=\frac{|\vec{b}|^{2}+|\vec{c}|^{2}-|\vec{a}|^{2}}{2|\vec{b}||\vec{c}|}=\frac{17}{28}$
Projection of $\overrightarrow{\mathrm{c}}$ on $\overrightarrow{\mathrm{b}}$
$=|\vec{c}| \cos \theta$
$=10 \times \frac{17}{28}$
$=\frac{85}{14}$
Click here to get exam-ready with eSaral
For making your preparation journey smoother of JEE, NEET and Class 8 to 10, grab our app now.
