Question:
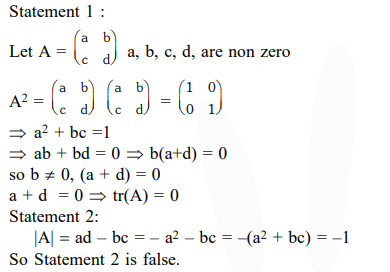
Let A be a $2 \times 2$ matrix with non-zero entries and let $\mathrm{A}^{2}=\mathrm{I}$, where $\mathrm{I}$ is $2 \times 2$ identity matrix. Define $\operatorname{Tr}(\mathrm{A})=$ sum of diagonal elements of $\mathrm{A}$ and $|\mathrm{A}|=$ determinant of matrix $\mathrm{A}$.
Statement-1: $\operatorname{Tr}(\mathrm{A})=0$.
Statement-2: $|\mathrm{A}|=1$.
Correct Option: , 3
Solution:
