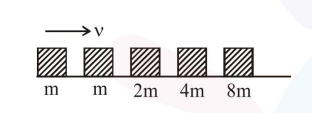
Blocks of masses $\mathrm{m}, 2 \mathrm{~m}, 4 \mathrm{~m}$ and $8 \mathrm{~m}$ are arranged in a line on a frictionless floor. Another block of mass $m$, moving with speed $v$ along the same line (see figure) collides with mass $\mathrm{m}$ in perfectly inelastic manner. All the subsequent collisions are also perfectly inelastic. By the time the last block of mass $8 \mathrm{~m}$ starts moving the total energy loss is $\mathrm{p} \%$ of the original energy. Value of ' $p$ ' is close to :
Correct Option: , 4
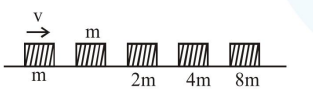
All collisions are perfectly inelastic, so after the final collision, all blocks are moving together. So let the final velocity be v', so on applying momentum conservation:
$\mathrm{mv}=16 \mathrm{~m} \mathrm{v}^{\prime} \Rightarrow \mathrm{v}^{\prime}=\mathrm{v} / 16$
Now initial energy $E_{i}=\frac{1}{2} m v^{2}$
Final energy : $\mathrm{E}_{\mathrm{f}}=\frac{1}{2} \times 16 \mathrm{~m} \times\left(\frac{\mathrm{v}}{16}\right)^{2}$
$\Rightarrow \mathrm{E}_{\mathrm{f}}=\frac{1}{2} \mathrm{~m} \frac{\mathrm{v}^{2}}{16}$
Energy loss : $\mathrm{E}_{\mathrm{i}}-\mathrm{E}_{\mathrm{f}}$
$\Rightarrow \frac{1}{2} \mathrm{mv}^{2}-\frac{1}{2} \mathrm{~m} \frac{\mathrm{v}^{2}}{16}$
$\Rightarrow \frac{1}{2} \mathrm{mv}^{2}\left[1-\frac{1}{16}\right] \Rightarrow \frac{1}{2} \mathrm{mv}^{2}\left[\frac{15}{16}\right]$
$\% \mathrm{p}=\frac{\text { Energy loss }}{\text { Original energy }} \times 100$
$=\frac{\frac{1}{2} \mathrm{mv}^{2}\left[\frac{15}{16}\right]}{\frac{1}{2} \mathrm{mv}^{2}} \times 100=93.75 \%$
$\Rightarrow$ Value of $P$ is close to 94 .
Click here to get exam-ready with eSaral
For making your preparation journey smoother of JEE, NEET and Class 8 to 10, grab our app now.
