With the usual notation, in $\triangle A B C$, if
$\angle \mathrm{A}+\angle \mathrm{B}=120^{\circ}, \mathrm{a}=\sqrt{3}+1$ and $\mathrm{b}=\sqrt{3}-1$
then the ratio $\angle \mathrm{A}: \angle \mathrm{B}$, is :
Correct Option: 1
$\mathrm{A}+\mathrm{B}=120^{\circ}$
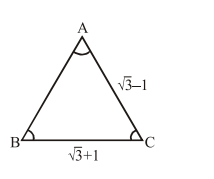
$\tan \frac{\mathrm{A}-\mathrm{B}}{2}=\frac{\mathrm{a}-\mathrm{b}}{\mathrm{a}+\mathrm{b}} \cot \left(\frac{\mathrm{C}}{2}\right)$
$=\frac{\sqrt{3}+1-\sqrt{3}+1}{2(\sqrt{3})} \cot \left(30^{\circ}\right)=\frac{1}{\sqrt{3}} \cdot \sqrt{3}=1$
$\frac{\mathrm{A}-\mathrm{B}}{2}=45^{\circ}$
$\begin{aligned} \Rightarrow \mathrm{A}-\mathrm{B} &=90^{\circ} \\ \mathrm{A}+\mathrm{B} &=120^{\circ} \end{aligned}$
$2 \mathrm{~A}=210^{\circ}$
$\mathrm{A}=105^{\circ}$
$\mathrm{B}=15^{\circ}$
$\therefore$ Option (1)
Click here to get exam-ready with eSaral
For making your preparation journey smoother of JEE, NEET and Class 8 to 10, grab our app now.
