Question:
Let $f: \mathrm{R} \rightarrow \mathrm{R}$ be defined as
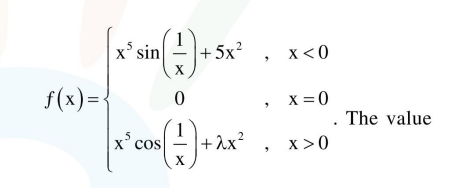
of $\lambda$ for which $f^{\prime \prime}(0)$ exists, is
Solution:
$f(\mathrm{x})=\mathrm{x}^{5} \cdot \sin \frac{1}{\mathrm{x}}+5 \mathrm{x}^{2} \quad$ if $\mathrm{x}<0$
$f(x)=0$ if $x=0$
$f(x)=x^{5} \cdot \cos \frac{1}{x}+\lambda x^{2} \quad$ if $x>0$
LHD of $f^{\prime}(\mathrm{x})$ at $\mathrm{x}=0$ is 10
RHD of $f^{\prime}(\mathrm{x})$ at $\mathrm{x}=0$ is $2 \lambda$
if $f^{\prime \prime}(0)$ exists then
$2 \lambda=10$
$\Rightarrow \lambda=5$
