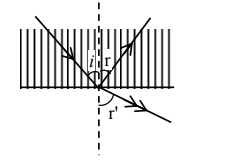
A ray of light passes from a denser medium to a rarer medium at an angle of incidence $i$. The reflected and refracted rays make an angle of $90^{\circ}$ with each other. The angle of reflection and refraction are respectively $r$ and $r^{\prime}$. The critical angle is given by :
Correct Option: , 4
$r+r^{\prime}+90^{\circ}=180^{\circ} \Rightarrow r^{\prime}=90-r=90-i$
$\mathrm{n}_{1} \sin \mathrm{i}=\mathrm{n}_{2} \sin \mathrm{r}^{\prime}=\mathrm{n}_{2} \sin (90-\mathrm{i})$
$n_{1} \sin i=n_{2} \cos i \Rightarrow \tan i=\frac{n_{2}}{n_{1}}$
Now $\sin C=\frac{n_{2}}{n_{1}}=\tan i$
$\Rightarrow \mathrm{C}=\sin ^{-1}(\tan \mathrm{i})=\sin ^{-1}(\tan \mathrm{r})$
Click here to get exam-ready with eSaral
For making your preparation journey smoother of JEE, NEET and Class 8 to 10, grab our app now.
