Let $\mathbb{C}$ be the set of all complex numbers. Let
$\mathrm{S}_{1}=\{\mathrm{z} \in \mathbb{C}:|\mathrm{z}-2| \leq 1\}$ and
$\mathrm{S}_{2}=\{\mathrm{z} \in \mathbb{C}: \mathrm{z}(1+\mathrm{i})+\overline{\mathrm{Z}}(1-\mathrm{i}) \geq 4\}$
Then, the maximum value of $\left|\mathrm{z}-\frac{5}{2}\right|^{2}$ for
$\mathrm{z} \in \mathrm{S}_{1} \cap \mathrm{S}_{2}$ is equal to :
Correct Option: , 4
$|\mathrm{t}-2| \leq 1 \quad$ Put $\mathrm{t}=\mathrm{x}+\mathrm{iy}$
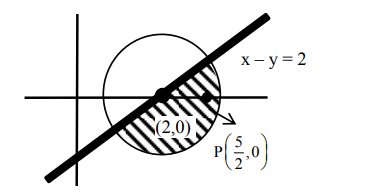
$(x-2)^{2}+y^{2} \leq 1$
Also, $\mathrm{t}(1+\mathrm{i})+\overline{\mathrm{t}}(1-\mathrm{i}) \geq 4$
Gives $x-y \geq 2$
Let point on circle be $\mathrm{A}(2+\cos \theta, \sin \theta)$
$\theta \in\left[-\frac{3 \pi}{4}, \frac{\pi}{4}\right]$
$(\mathrm{AP})^{2}=\left(2+\cos \theta-\frac{5}{2}\right)^{2}+\sin ^{2} \theta$
$=\cos ^{2} \theta-\cos \theta+\frac{1}{4}+\sin ^{2} \theta$
$=\frac{5}{4}-\cos \theta$
For $(\mathrm{AP})^{2}$ maximum $\theta=-\frac{3 \pi}{4}$
$(\mathrm{AP})^{2}=\frac{5}{4}+\frac{1}{\sqrt{2}}=\frac{5 \sqrt{2}+4}{4 \sqrt{2}}$