Question.
State which pairs of triangles in figure, are similar. Write the similarity criterion used by you for answering the question and also write the pairs of similar triangles in the symbolic form:
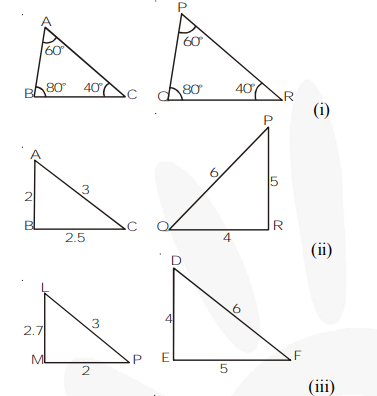
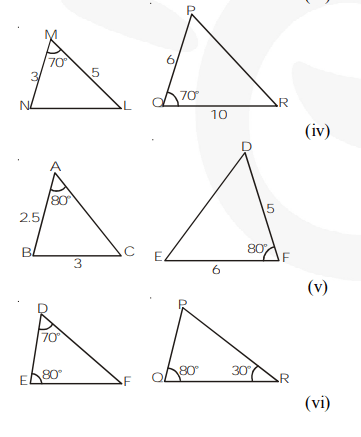
State which pairs of triangles in figure, are similar. Write the similarity criterion used by you for answering the question and also write the pairs of similar triangles in the symbolic form:
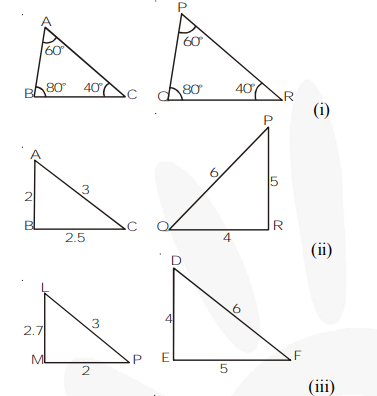

Solution:
(i) Yes. $\angle \mathrm{A}=\angle \mathrm{P}=60^{\circ}, \angle \mathrm{B}=\angle \mathrm{Q}=80^{\circ}$,
$\angle \mathrm{C}=\angle \mathrm{R}=40^{\circ}$
Therefore, $\Delta \mathrm{ABC} \sim \Delta \mathrm{PQR}$.
By AAA similarity criterion
(ii) Yes.
$\frac{A B}{Q R}=\frac{2}{4}=\frac{1}{2}, \frac{B C}{R P}=\frac{2.5}{5}=\frac{1}{2}, \frac{C A}{P Q}=\frac{3}{6}=\frac{1}{2}$
Therefore, $\Delta \mathrm{ABC} \sim \Delta \mathrm{QRP}$.
By SSS similarity criterion.
(iii) No.
$\frac{\mathrm{MP}}{\mathrm{DE}}=\frac{2}{4}=\frac{1}{2}, \frac{\mathrm{LP}}{\mathrm{DF}}=\frac{3}{6}=\frac{1}{2}, \frac{\mathrm{LM}}{\mathrm{EF}}=\frac{2.7}{5} \neq \frac{1}{2}$
i.e., $\frac{M P}{D E}=\frac{L P}{D F} \neq \frac{L M}{E F}$
Thus, the two triangles are not similar.
(iv) Yes,
$\frac{M N}{O P}=\frac{M L}{O R}=\frac{1}{2}$
and $\angle \mathrm{NML}=\angle \mathrm{PQR}=70^{\circ}$
By SAS similarity criterion
$\Delta \mathrm{NML} \sim \Delta \mathrm{PQR}$
(v) No,
$\frac{A B}{F D} \neq \frac{A C}{F E}$
Thus, the two triangles are not similar
(vi) In triangle DEF $\angle \mathrm{D}+\angle \mathrm{E}+\angle \mathrm{F}=180^{\circ}$
$70^{\circ}+80^{\circ}+\angle \mathrm{F}=180^{\circ}$
$\angle \mathrm{F}=30^{\circ}$
In triangle PQR
$\angle \mathrm{P}+80^{\circ}+30^{\circ}=180^{\circ}$
$\angle \mathrm{P}=70^{\circ}$
$\angle \mathrm{E}=\angle \mathrm{Q}=80^{\circ}$
$\angle \mathrm{D}=\angle \mathrm{P}=70^{\circ}$
$\angle \mathrm{r}=\angle \mathrm{K}=30$
By AAA similarity criterion
$\Delta \mathrm{DEF} \sim \Delta \mathrm{PQR}$
(i) Yes. $\angle \mathrm{A}=\angle \mathrm{P}=60^{\circ}, \angle \mathrm{B}=\angle \mathrm{Q}=80^{\circ}$,
$\angle \mathrm{C}=\angle \mathrm{R}=40^{\circ}$
Therefore, $\Delta \mathrm{ABC} \sim \Delta \mathrm{PQR}$.
By AAA similarity criterion
(ii) Yes.
$\frac{A B}{Q R}=\frac{2}{4}=\frac{1}{2}, \frac{B C}{R P}=\frac{2.5}{5}=\frac{1}{2}, \frac{C A}{P Q}=\frac{3}{6}=\frac{1}{2}$
Therefore, $\Delta \mathrm{ABC} \sim \Delta \mathrm{QRP}$.
By SSS similarity criterion.
(iii) No.
$\frac{\mathrm{MP}}{\mathrm{DE}}=\frac{2}{4}=\frac{1}{2}, \frac{\mathrm{LP}}{\mathrm{DF}}=\frac{3}{6}=\frac{1}{2}, \frac{\mathrm{LM}}{\mathrm{EF}}=\frac{2.7}{5} \neq \frac{1}{2}$
i.e., $\frac{M P}{D E}=\frac{L P}{D F} \neq \frac{L M}{E F}$
Thus, the two triangles are not similar.
(iv) Yes,
$\frac{M N}{O P}=\frac{M L}{O R}=\frac{1}{2}$
and $\angle \mathrm{NML}=\angle \mathrm{PQR}=70^{\circ}$
By SAS similarity criterion
$\Delta \mathrm{NML} \sim \Delta \mathrm{PQR}$
(v) No,
$\frac{A B}{F D} \neq \frac{A C}{F E}$
Thus, the two triangles are not similar
(vi) In triangle DEF $\angle \mathrm{D}+\angle \mathrm{E}+\angle \mathrm{F}=180^{\circ}$
$70^{\circ}+80^{\circ}+\angle \mathrm{F}=180^{\circ}$
$\angle \mathrm{F}=30^{\circ}$
In triangle PQR
$\angle \mathrm{P}+80^{\circ}+30^{\circ}=180^{\circ}$
$\angle \mathrm{P}=70^{\circ}$
$\angle \mathrm{E}=\angle \mathrm{Q}=80^{\circ}$
$\angle \mathrm{D}=\angle \mathrm{P}=70^{\circ}$
$\angle \mathrm{r}=\angle \mathrm{K}=30$
By AAA similarity criterion
$\Delta \mathrm{DEF} \sim \Delta \mathrm{PQR}$
Click here to get exam-ready with eSaral
For making your preparation journey smoother of JEE, NEET and Class 8 to 10, grab our app now.
