Question:
The area bounded by the lines $y=|| x-1|-2|$ is
Note: NTA has dropped this question in the final official answer key.
Solution:
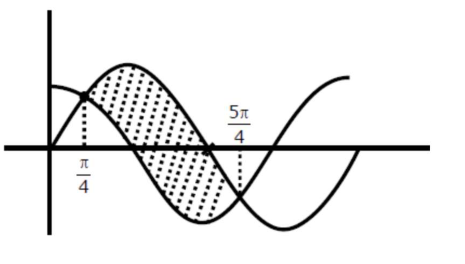
$\mathrm{A}=\int_{\frac{\pi}{4}}^{\frac{5 \pi}{4}}(\sin \mathrm{x}-\cos \mathrm{x}) \mathrm{dx}=[-\cos \mathrm{x}-\sin \mathrm{x}]_{\pi / 4}^{5 \pi / 4}$
$=-\left[\left(\cos \frac{5 \pi}{4}+\sin \frac{\pi}{4}\right)-\left(\cos \frac{\pi}{4}+\sin \frac{\pi}{4}\right)\right]$
$=-\left[\left(-\frac{1}{\sqrt{2}}-\frac{1}{\sqrt{2}}\right)-\left(\frac{1}{\sqrt{2}}+\frac{1}{\sqrt{2}}\right)\right]$
$=\frac{4}{\sqrt{2}}=2 \sqrt{2}$
$\Rightarrow \mathrm{A}^{4}=(2 \sqrt{2})^{4}=64$
