The boat goes 30 km upstream and 44 km downstream in 10 hours.

Let the speed of the boat in still water be x km/hr and the speed of the stream be y km/hr

Speed upstream $=(x-y) \mathrm{km} / \mathrm{hr}$

Speed down stream $=(x+y) \mathrm{km} / \mathrm{hr}$

Now,

Time taken to cover $30 \mathrm{~km}$ upstream $=\frac{30}{x-y}$ hrs

Time taken to cover $44 \mathrm{~km}$ down stream $=\frac{44}{x+y}$ hrs

But total time of journey is 10 hours

$\frac{30}{x-y}+\frac{44}{x+y}=10 \cdots(i)$

Time taken to cover $40 \mathrm{~km}$ upstream $=\frac{40}{x-y} h r s$

Time taken to cover $55 \mathrm{~km}$ down stream $=\frac{55}{x+y} h r s$

In this case total time of journey is given to be 13 hours

Therefore, $\frac{40}{x-y}+\frac{55}{x+y}=13$$\ldots$ (ii)

Putting $\frac{1}{x-y}=u$ and $\frac{1}{x+y}=v$ in equation $(i)$ and $(i i)$ we get

$30 u+44 v=10$

$40 u+55 v=10$

$30 u+44 v-10=0 \cdots(i i i)$

$40 u+55 v-13=0 \cdots(i v)$

Solving these equations by cross multiplication we get

$\frac{u}{44 \times-13-55 \times-10}=\frac{-v}{30 \times-13-40 \times-10}=\frac{1}{30 \times 55-40 \times 44}$

$\frac{u}{-572+550}=\frac{-v}{-390+400}=\frac{1}{1650-1760}$

$\frac{u}{-22}=\frac{-v}{10}=\frac{1}{-110}$

$u=\frac{2}{10}$ and $v=\frac{1}{11}$

Now,

$u=\frac{2}{10}$

$\frac{1}{x-y}=\frac{2}{10}$

$1 \times 10=2(x-y)$

$10=2 x-2 y \div 2$

$5=x-y \cdots(v)$

$v=\frac{1}{11}$

$\frac{1}{x+y}=\frac{1}{11}$

$1 \times 11=1(x+y)$

$11=x+y \cdots(v i)$

By solving equation $(v)$ and $(v i)$ we get,

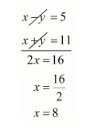
Substituting $x=8$ in equation $(v i)$ we get,

$x+y=11$

$8+y=11$

$y=11-8$

$y=3$

Hence, speed of the boat in still water is $8 \mathrm{~km} / \mathrm{hr}$

Speed of the stream is $3 \mathrm{~km} / \mathrm{hr}$