The diagonal of a rectangular field is 60 metres more than the shorter side.

Solution:

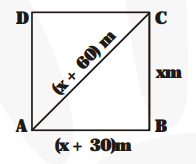
In rectangle ABCD, let the shorter side BC = x metres.

Then AB = (x + 30) metres and diagonal

AC = (x + 60) metres.

By Pythagoras Theorem we have



$\mathrm{AB}^{2}+\mathrm{BC}^{2}=\mathrm{AC}^{2}$

$\Rightarrow(x+30)^{2}+x^{2}$

$=(x+60)^{2}$

$\Rightarrow x^{2}+60 x+900+x^{2}$

$=x^{2}+120 x+3600$

$\Rightarrow x^{2}-60 x-2700=0$

$a=1, b=-60, c=-2700$

$D=(-60)^{2}-4 \times 1 \times(-2700)$

$=3600+10800=14400$

$\Rightarrow \sqrt{10}=\sqrt{14400}=120$

Then $x=\frac{-\mathbf{b} \pm \sqrt{\mathbf{D}}}{\mathbf{2 a}}=\frac{\mathbf{6 0} \pm \mathbf{1 2 0}}{\mathbf{2}}=90,-30$

We reject $x=-30 \quad(\because x+0)$

$\Rightarrow x=90$

$\Rightarrow \mathrm{BC}=90 \mathrm{~m}$ and $\mathrm{AB}=(90+30) \mathrm{m}=120 \mathrm{~m}$

Hence, the sides of the rectangular field are $90 \mathrm{~m}$ and $120 \mathrm{~m}$.