Question:
The diameters of circles (in mm) drawn in a design are given below:
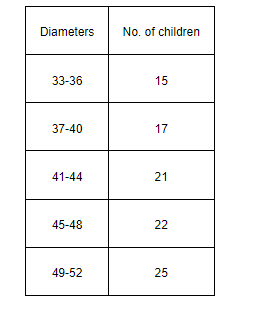
Solution:
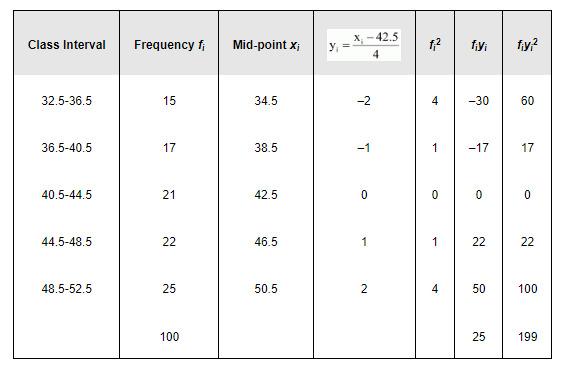
Here, N = 100, h = 4
Let the assumed mean, A, be 42.5.
Mean, $\bar{x}=A+\frac{\sum_{i=1}^{5} f_{i} y_{i}}{N} \times h=42.5+\frac{25}{100} \times 4=43.5$
$\operatorname{Variance}\left(\sigma^{2}\right)=\frac{h^{2}}{N^{2}}\left[N \sum_{i=1}^{3} f_{i} y_{i}^{2}-\left(\sum_{i=1}^{3} f_{i} y_{i}\right)^{2}\right]$
$=\frac{16}{10000}\left[100 \times 199-(25)^{2}\right]$
$=\frac{16}{10000}[19900-625]$
$=\frac{16}{10000} \times 19275$
$=30.84$
$\therefore$ Stan dard deviation $(\sigma)=5.55$
Click here to get exam-ready with eSaral
For making your preparation journey smoother of JEE, NEET and Class 8 to 10, grab our app now.
