Question:
The difference between the radii of $3^{\mathrm{rd}}$ and $4^{\text {th }}$ orbits of $\mathrm{Li}^{2+}$ is $\Delta \mathrm{R}_{1}$. The difference between the radii of $3^{\mathrm{rd}}$ and $4^{\text {th }}$ orbits of $\mathrm{He}^{+}$is $\Delta \mathrm{R}_{2}$. Ratio $\Delta \mathrm{R}_{1}: \Delta \mathrm{R}_{2}$ is :
Correct Option: , 3
Solution:
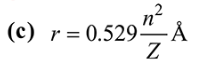
For $\mathrm{Li}^{2+}$
$\left(r_{\mathrm{Li}^{2+}}\right)_{n=4}-\left(r_{\mathrm{Li}^{2+}}\right)_{n=3}=\frac{0.529}{3}\left[4^{2}-3^{2}\right]=\Delta R_{1}$
For $\mathrm{He}^{+}$,
$\left(r_{\mathrm{He}^{+}}\right)_{n=4}-\left(r_{\mathrm{He}^{+}}\right)_{n=3}=\frac{0.529}{2}\left[4^{2}-3^{2}\right]=\Delta R_{2}$
$\frac{\Delta R_{1}}{\Delta R_{2}}=\frac{2}{3}$