The dimensions of a rectangle ABCD are 51 cm x 25 cm. A trapezium PQCD with its parallel sides QC and PD in the ratio 9 : 8, is cut off from the
rectangle as shown in the figure. If the area of the trapezium PQCD is 5/6 th part of the area of the rectangle. Find the lengths QC and PD.
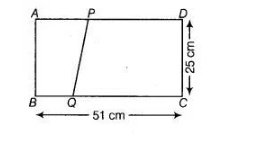
We have dimensions of a recstangle $A B C D$ as $51 \mathrm{~cm}$ and $25 \mathrm{~cm}$. Also, in trapezium $P Q C D$, parallel sides $Q C$ and $P D$ are in the ratio $9: 8$.
i.e.n $Q C: P D=9: 8$
Let, length $Q C=9 x$
and $P D=8 x$
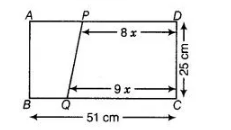
According to the question,
Area of trapezium $P Q C D=\frac{5}{6}$ area of rectangle $A B C D$
$\Rightarrow \frac{1}{2}$ (Sum of parallel sides) $\times$ Distance between parallel sides $=\frac{5}{6} \times(B C \times C D)$
$\Rightarrow \quad \frac{1}{2}(8 x+9 x) \times 25=\frac{5}{6} \times 51 \times 25$
$\Rightarrow \quad \frac{1}{2} \times 17 x \times 25=\frac{5}{6} \times 51 \times 25$
$\Rightarrow \quad x=\frac{5 \times 51 \times 25 \times 2}{25 \times 17 \times 6}$
$\Rightarrow \quad x=5$
$\therefore$ Lengths, $\quad Q C=9 \times 5=45 \mathrm{~cm}$
and $\quad P D=8 \times 5=40 \mathrm{~cm}$.