The displacement time graph of a particle executing S.H.M. is given in figure : (sketch is schematic and not to scale)
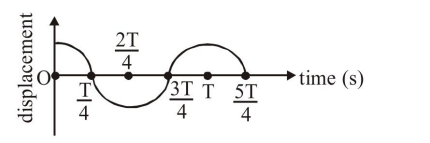
Which of the following statements is/are true for this motion ?
(A) The force is zero $\mathrm{t}=\frac{3 \mathrm{~T}}{4}$
(B) The acceleration is maximum at $\mathrm{t}=\mathrm{T}$
(C) The speed is maximum at $\mathrm{t}=\frac{\mathrm{T}}{4}$
(D) The P.E. is equal to $\mathrm{K}$.E. of the oscillation
at $\mathrm{t}=\frac{\mathrm{T}}{2}$
Correct Option: , 4
(A) $F=m a$
$a=-\omega^{2} X$
at $\frac{3 \mathrm{~T}}{4}$ displacement zero $(\mathrm{x}=0)$, so $\mathrm{a}=0$
$F=0$
(B) at $\mathrm{t}=\mathrm{T}$
displacement $(x)=A$
$\mathrm{x}$ maximum, So acceleration is maximum.
(C) $\mathrm{V}=\omega \sqrt{\mathrm{A}^{2}-\mathrm{x}^{2}}$
$\mathrm{V}_{\max }$ at $\mathrm{x}=0$
$\mathrm{~V}_{\max }=\mathrm{A} \omega$
at $\mathrm{t}=\frac{\mathrm{T}}{4}, \quad \mathrm{x}=0, \quad$ So $\mathrm{V}_{\max }$
(D) $\mathrm{KE}=\mathrm{PE}$
$\therefore$ at $x=\frac{A}{\sqrt{2}}$
at $\mathrm{t}=\frac{\mathrm{T}}{2} \quad \mathrm{x}=-\mathrm{A} \quad$ (So not possible)