The first ionization constant of H2­­S is 9.1 × 10–8. Calculate the concentration

Solution:

(i) To calculate the concentration of HS– ion:

Case I (in the absence of HCl):

Let the concentration of HS– be x M.

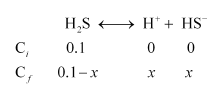
Then, $K_{a_{1}}=\frac{\left[\mathrm{H}^{+}\right]\left[\mathrm{HS}^{-}\right]}{\left[\mathrm{H}_{2} \mathrm{~S}\right]}$

$9.1 \times 10^{-8}=\frac{(x)(x)}{0.1-x}$

$\left(9.1 \times 10^{-8}\right)(0.1-x)=x^{2}$

Taking $0.1-x \mathrm{M} ; 0.1 \mathrm{M}$, we have $\left(9.1 \times 10^{-8}\right)(0.1)=x^{2}$.

$9.1 \times 10^{-9}=x^{2}$

$x=\sqrt{9.1 \times 10^{-9}}$

$=9.54 \times 10^{-5} \mathrm{M}$

$\Rightarrow\left[\mathrm{HS}^{-}\right]=9.54 \times 10^{-5} \mathrm{M}$

Case II (in the presence of HCl):

In the presence of $0.1 \mathrm{M}$ of $\mathrm{HCl}$, let $\left[\mathrm{HS}^{-}\right]$be $y \mathrm{M}$.

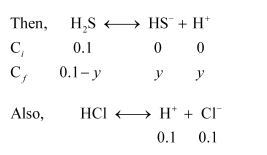
Now, $K_{a_{1}}=\frac{\left[\mathrm{HS}^{-}\right]\left[\mathrm{H}^{+}\right]}{\left[\mathrm{H}_{2} \mathrm{~S}\right]}$

$K_{a_{1}}=\frac{[y](0.1+y)}{(0.1-y)}$

$9.1 \times 10^{-8}=\frac{y \times 0.1}{0.1}$        $(\because 0.1-y ; 0.1 \mathrm{M})$

$9.1 \times 10^{-8}=y$                                            (and $0.1+y ; 0.1 \mathrm{M}$ )

$\Rightarrow\left[\mathrm{HS}^{-}\right]=9.1 \times 10^{-8}$

(ii) To calculate the concentration of $\left[\mathrm{S}^{2-}\right]$ :

Case I (in the absence of 0.1 M HCl):

$\mathrm{HS}^{-} \longleftrightarrow \mathrm{H}^{+}+\mathrm{S}^{2-}$

$\left[\mathrm{HS}^{-}\right]=9.54 \times 10^{-5} \mathrm{M}$ (From first ionization, case $\mathrm{I}$ )

Let $\left[\mathrm{S}^{2-}\right]$ be $X$.

Also, $\left[\mathrm{H}^{+}\right]=9.54 \times 10^{-5} \mathrm{M}$ (From first ionization, case $\mathrm{l}$ )

$K_{a_{2}}=\frac{\left[\mathrm{H}^{+}\right]\left[\mathrm{S}^{2-}\right]}{\left[\mathrm{HS}^{-}\right]}$

$K_{a_{2}}=\frac{\left(9.54 \times 10^{-5}\right)(X)}{9.54 \times 10^{-5}}$

$1.2 \times 10^{-13}=X=\left[\mathrm{S}^{2-}\right]$

Case II (in the presence of 0.1 M HCl):

Again, let the concentration of $\mathrm{HS}^{-}$be $X^{\prime} \mathrm{M}$.

$\left[\mathrm{HS}^{-}\right]=9.1 \times 10^{-8} \mathrm{M}$ (From first ionization, case II)

$\left[\mathrm{H}^{+}\right]=0.1 \mathrm{M}($ From $\mathrm{HCl}$, case $\|$ )

$\left[\mathrm{S}^{2-}\right]=X^{\prime}$

Then, $K_{a_{2}}=\frac{\left[\mathrm{H}^{+}\right]\left[\mathrm{S}^{2-}\right]}{\left[\mathrm{HS}^{-}\right]}$

$1.2 \times 10^{-13}=\frac{(0.1)\left(X^{\prime}\right)}{9.1 \times 10^{-8}}$

$10.92 \times 10^{-21}=0.1 X^{\prime}$

$\frac{10.92 \times 10^{-21}}{0.1}=X^{\prime}$

$X^{\prime}=\frac{1.092 \times 10^{-20}}{0.1}$

$=1.092 \times 10^{-19} \mathrm{M}$

$\Rightarrow K_{a_{1}}=1.74 \times 10^{-5}$