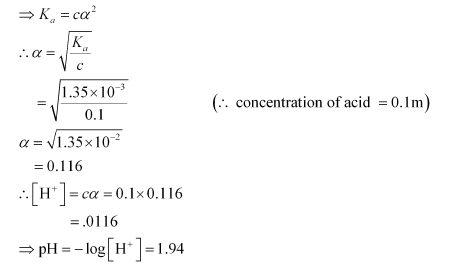
The ionization constant of chloroacetic acid is 1.35 × 10–3.

Solution:

It is given that $\mathrm{K}_{a}$ for $\mathrm{ClCH}_{2} \mathrm{COOH}$ is $1.35 \times 10^{-3}$.

$\mathrm{ClCH}_{2} \mathrm{COONa}$ is the salt of a weak acid i.e., $\mathrm{ClCH}_{2} \mathrm{COOH}$ and a strong base i.e., $\mathrm{NaOH}$.

$\mathrm{ClCH}_{2} \mathrm{COO}^{-}+\mathrm{H}_{2} \mathrm{O} \longleftrightarrow \mathrm{ClCH}_{2} \mathrm{COOH}+\mathrm{OH}^{-}$

$K_{h}=\frac{\left[\mathrm{ClCH}_{2} \mathrm{COOH}\right]\left[\mathrm{OH}^{-}\right]}{\left[\mathrm{ClCH}_{2} \mathrm{COO}^{-}\right]}$

$K_{h}=\frac{K_{w}}{K_{a}}$

$K_{h}=\frac{10^{-14}}{1.35 \times 10^{-3}}$

$=0.740 \times 10^{-11}$

Also, $K_{h}=\frac{x^{2}}{0.1}$

(where $x$ is the concentration of $\mathrm{OH}^{-}$and $\mathrm{ClCH}_{2} \mathrm{COOH}$ )

$0.740 \times 10^{-11}=\frac{x^{2}}{0.1}$

$0.074 \times 10^{-11}=x^{2}$

$\Rightarrow x^{2}=0.74 \times 10^{-12}$

$x=0.86 \times 10^{-6}$

$\left[\mathrm{OH}^{-}\right]=0.86 \times 10^{-6}$

$\therefore\left[\mathrm{H}^{+}\right]=\frac{K_{w}}{0.86 \times 10^{-6}}$

$=\frac{10^{-14}}{0.86 \times 10^{-6}}$

$\left[\mathrm{H}^{+}\right]=1.162 \times 10^{-8}$

$\mathrm{pH}=-\log \left[\mathrm{H}^{+}\right]$

$=7.94$