The length of the hypotenuse of an isosceles right triangle whose one side is 42 cm is
(a) 12 cm
(b) 8 cm
(c) 82 cm
(d) 122 cm
Given: One side of isosceles right triangle is 4√2cm
To find: Length of the hypotenuse.
We know that in isosceles triangle two sides are equal.
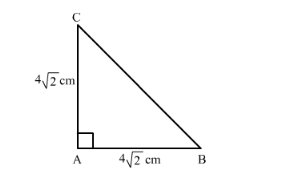
In isosceles right triangle $\mathrm{ABC}$, let $\mathrm{AB}$ and $\mathrm{AC}$ be the two equal sides of measure $4 \sqrt{2} \mathrm{~cm}$.
Applying Pythagoras theorem, we get
$\mathrm{BC}^{2}=\mathrm{AB}^{2}+\mathrm{AC}^{2}$
$\mathrm{BC}^{2}=(4 \sqrt{2})^{2}+(4 \sqrt{2})^{2}$
$\mathrm{BC}^{2}=32+32$
$\mathrm{BC}^{2}=64$
$B C=8 \mathrm{~cm}$
Hence correct answer is (b)
Click here to get exam-ready with eSaral
For making your preparation journey smoother of JEE, NEET and Class 8 to 10, grab our app now.
