The mass density of a spherical galaxy varies
as $\frac{\mathrm{K}}{\mathrm{r}}$ over a large distance ' $\mathrm{r}$ ' from its centre.
In that region, a small star is in a circular orbit of radius $R$. Then the period of revolution, $T$ depends on $R$ as :
Correct Option: , 3
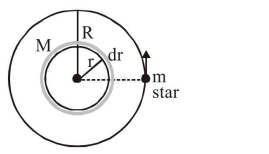
$\mathrm{dm}=\rho \mathrm{dv}$
$\mathrm{dm}=\left(\frac{\mathrm{k}}{\mathrm{r}}\right)\left(4 \pi \mathrm{r}^{2} \mathrm{dr}\right)$
$\mathrm{dm}=4 \pi \mathrm{krdr}$
$\mathrm{M}=\int_{0}^{\mathrm{R}} \mathrm{dm}=\int_{0}^{\mathrm{R}} 4 \pi \mathrm{krdr}$
$\mathrm{M}=\left.4 \pi \mathrm{k} \frac{\mathrm{r}^{2}}{2}\right|_{0} ^{\mathrm{R}}$
$\mathrm{M}=2 \pi \mathrm{k}\left(\mathrm{R}^{2}-0\right)$
$\mathrm{M}=2 \pi \mathrm{kR}^{2}$
for circular motion gravitational force will provide required centripital force or
$\frac{\mathrm{GMm}}{\mathrm{R}^{2}}=\frac{\mathrm{mv}^{2}}{\mathrm{R}}$
$\frac{\mathrm{G}\left(2 \pi \mathrm{kR}^{2}\right) \mathrm{m}}{\mathrm{R}^{2}}=\frac{\mathrm{mv}^{2}}{\mathrm{R}} \Rightarrow \mathrm{v}=\sqrt{2 \pi \mathrm{GkR}}$
Time period $T=\frac{2 \pi R}{v}$
$\mathrm{T}=\frac{2 \pi \mathrm{R}}{\sqrt{2 \pi \mathrm{GkR}}} \propto \sqrt{\mathrm{R}}$
or $\mathrm{T}^{2} \propto \mathrm{R}$
