Question:
The natural number $\mathrm{m}$, for which the coefficient of $x$ in the binomial expansion of $\left(x^{m}+\frac{1}{x^{2}}\right)^{22}$
is 1540 , is___________.
Solution:
$\mathrm{T}_{\mathrm{r}+1}={ }^{22} \mathrm{C}_{\mathrm{r}}\left(\mathrm{x}^{\mathrm{m}}\right)^{22-\mathrm{r}}\left(\frac{1}{\mathrm{x}^{2}}\right)^{\mathrm{r}}={ }^{22} \mathrm{C}_{\mathrm{r}} \mathrm{x}^{22 \mathrm{~m}-\mathrm{mr}-2 \mathrm{r}}$
$={ }^{22} \mathrm{C}_{\mathrm{r}} \mathrm{x}$
$\because{ }^{22} \mathrm{C}_{3}=22 \mathrm{C}_{19}=1540$
$\therefore r=3$ or 19
$22 m-m r-2 r=1$
$\mathrm{m}=\frac{2 \mathrm{r}+1}{22-5}$
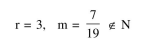
$r=19, m=\frac{38+1}{22-19}=\frac{39}{3}=13$
$\mathrm{m}=13$
